Definition: Compound interest is a great thing when you are earning it! Compound interest is your investment earns an interest on both the principal (the original amount of money) and the interest the investment has already earned. Simply put in mandarin is "连本带利,利上加利", at your advantage.

What’s the value of power of compounding.
This is one of the most valuable Financial Literacy that you will appreciate in terms of investment. Basically, this is what we call money grow money or make your money work harder for you. Imagine when you gained a 4.5% interest per annum from your investment with $100,000, on the first year you will have $104,500. With the effect of compounding interest, the next year 4.5% interest rate will actually apply onto your compounded amount of $104,500 instead of your base amount of $100,000. In this case, it will be $109,202.50, and so on and so forth. However, for the power of compounding to proof it’s potential on the returns, we will need a long investment period.
Let’s stretch the above example to a 20 years period. So from the chart below, you can see that the value has exponentially increased from $100,000 to $241,171, that is more than 141% return!
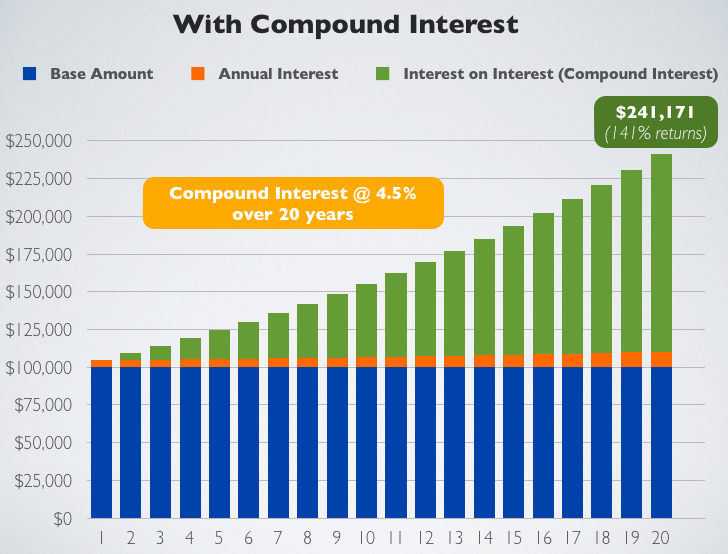
Let’s see how will it look if there is no effect of compounding interest.
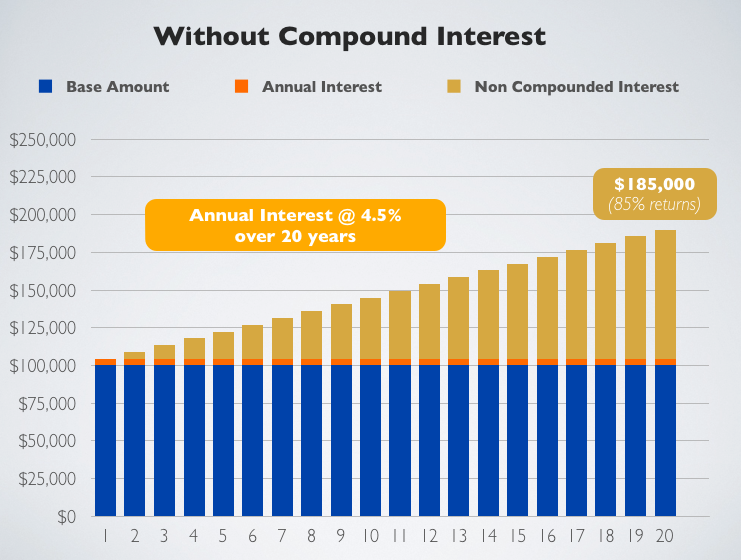
As you can see, the returns without compounding interest is merely only 85% over 20 years. As compared to the first chart above with compounding interest, there is a 56% ($56,000) difference. Ouch!
Rule of 72
The Rule of 72 is a simplified way to determine how long an investment will take to double, given a fixed annual rate of interest with the effect of compounding interest. By dividing 72 by the annual rate of return, investors can get a rough estimate of how many years it will take for the initial investment to duplicate itself.Rate of Return in Years = 72 / Interest Rate.
72/4.5 = 16 years for your invested money to double.
If you investment $100,000 with an a constant interest rate of 4.5% per annum, it will approximately takes 16 years for it to double to $200,000. The lower the interest rate the longer the rate of returns.
So if you need an estimation on your investment returns, Rule of 72 can be useful.
Formula
If you want to have a more accurate way to know and plan your investment. Here is the formula for compound interest:
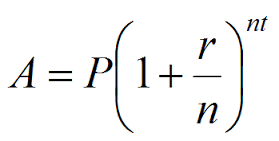
- A: The total compounded amount.
- P: The principal or base amount.
- r: Interest rate
- n: The number of times per years the interest is compounded.
- t: number of years.
If you have an investment with principal of $100,000, and the interest compounds once a year at an interest rate of 4.5%, how much money do you have in your account at the end of 20 years?
A = $100,000 (1+ (0.045/1) 1 x 20
A = $100,000 (1.045) 20
A = $100,000 (2.411714025)
A = $241,171
So if you have understand the power of compounding, always use it to your advantage. For investment it will definitely be a huge advantage. However, in terms of certain loans when compounding interest takes effect on it, I’m certain that you will be paying significantly more than what you have loaned.
